Vibration (SHM equation)

 

Homework Vibration

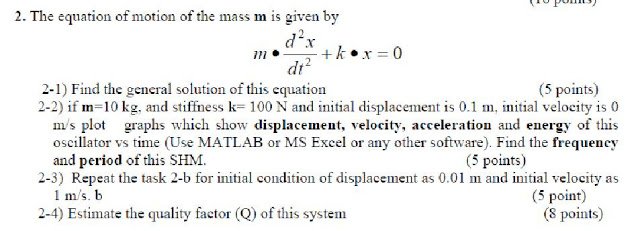
Answer

1.

The general REAL solutions of the UN DAMPED elastic oscillator are

$x(t) =A*cos(2*pi*t/T)$, where x is the position versus time t and T is the period of the oscillator

$T =2*pi*sqrt(m/k)$

$x(t) =A*cos(sqrt{(k/m)}*t)$

2.

$v(t) = x'(t) =A*sqrt{(k/m)}*sin(sqrt{(k/m)}*t)$

$a(t) = v'(t) =-A *k/m*cos(sqrt{(k/m)}*t))$

$A =0.1 m$ , $m=10 kg$, $k=100 N$

For the graphs see attached file no.1

$x =0.1*cos(3.16*t)$

$v =0.316*sin(3.16*t)$

$a =-1*cos(3.16*t)$

3.

$A =0.01 m$, $V0 =1 m/s$

$0.01*sqrt{(k/m)} = 1$    $sqrt{(k/m)} =100$,  $k/m = 10^4$
$x =0.01*cos(10^4*t)$

$v = 1*sin(10^4 *t)$

$a = -100*cos(10^4 *t)$

for the graph see attached file no 2

4.

Since there is no DUMPING (there are no mechanical losses of the energy of the oscillator) the friction force is null (Ff =0).

 The quality factor Q is proportional to

$Q =1/Ff *sqrt{(m/k)} = infty$