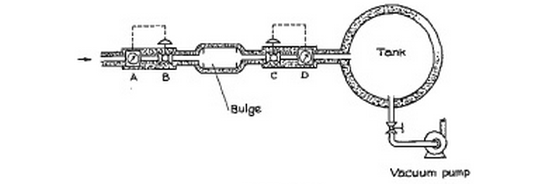
Steady air flow (bulge and tank)

A well-insulated pipe of 2.54 cm inside diameter carries air at 2 bar pressure and 366.5 K. It is connected to a $0.0283 m^3$ insulated bulge as shown in Figure P3.12.

The air in the bulge is initially at one bar pressure and 311 K. A and D are flow meters which accurately measure the mass rate of airflow. Valves B and C control the airflow into and out of the bulge.Connected to the bulge is $0.283 m^3$ rigid, adiabatic tank which is initially evacuated to a very low pressure.

At the start of the operation. valve B is opened to allow 4.54 g/s of air flow into the bulge; simultaneously, valve C is operated to transfer exactly 4.54 g/s: from the bulge into the tank. These flows are maintained constant and are measured by the mass flow meters. Air may be assumed to be an ideal gas with a constant $C_p$  of $29.3 J/(mol*K)$. Assume also that the gases both in the bulge and in large tank are completely mixed so that there are no temperature or pressure gradients present.

(a) What is the temperature and pressure of the air in the bulge after 6 s?

(b) What is the temperature and pressure of the gas in the large tank after 3 s?

a)

Air that enters the bulge with temperature $T1 =366.5 K$ heats the air inside until temperature $T x$.

Given heat is $Q =N1*C*(T1-T x)$ where C is the specific heat of the air and $N1$ is the number of moles of air that enters the bulge.

Air inside the bulge is heated from $T0=311 K$ to $T x$: $Q =(N+N1-N2)*C*(T x –T0)$

Where N is the number of air moles initially inside the bulge N1 is the entering number of moles of air, N2 is the exiting number of moles of air.

But since the molar mass of air $M=28.97 g/mol$ is constant independent of temperature, it results $N1 =N2$ (the air flow mass is the same inside and outside).

$N1/t =N2/t=(m/t)/M =4.54/28.97 =0.1567 mol/sec$

After 6 seconds $N1 =0.1567*6 =0.94 mol$

Therefore (from heat equality)

$N1*C*(T1-T x) =N*C*(T x-T0)$

$N1*T1 –N1*T x =N*T x –N*T0$

$T x*(N+N1) =N1*T1 +N*T0$

$T x = (N1*T1 +N*T0)/(N+N1)$

We know $T1 =366.5 K$, $T0=311 K$.

For the air inside the bulge initially we can write:

$P0*V0= N*R*T0$

Here $P0 =1 bar = 10^5 N/m^2$, $R=8.31 J/mol$, $T0 =311 K$ so that

$N =P0*V0/(R*T0) =1.095 mol$

Thus

$T x =(0.94*366.5 +1.095*311)/(0.94+1.095) =336.63 K$

b)

Since there are no temperature gradients the temperature of the gas inside the tank after 3 seconds is the same as the temperature of the gas exiting the bulge after 3 seconds. Pressure inside the tank is different because of the airflow regulator C)

$N2’=N1’ =3*0.1567 =0.4701 mole$

$T2=Tx’ = (0.47*366.5 +1.095*311)/(0.47+1.095) =327.7 K$

The pressure in the larger tank after 3 seconds can be found from the general equation of gases:

$P2 = N2’*R*T2/V2 =0.47*8.31*327.7/0.283 =4522.6 N/m^2$