Parallel charged wires
Charged wires
You are given two parallel wires, charged with the same charge in absolute value, one is positively and the other is negatively charged.
a. Find the electric field at all points between the charged wires in the plane xy.
b. What is the potential difference between the two wires.
c. Please find the capacitance per unit length of this system.
d. Find the minimum spacing between the charged wires if the potential is given $V$, so that the electric field is less than the maximum electric field strength of air $Eb$.
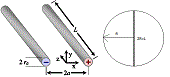
Electric field
a)
Consider that you have only one wire having a linear charge distribution $\lambda$.
We choose a Gauss spherical surface of radius $R=L/2$ that has inside it exactly the entire wire length $L$ as in the figure.
We then apply the Gauss law (electric flux through closed surface=charge inside the surface/permittivity):
$\iint E(r)dS=Q(inside)/\epsilon0$ $S=4\pi R^2$
$Q(inside)=\lambda L=2R\lambda$
$4\pi R^2*E(R)=2R\lambda/\epsilon0$
Therefore the field of a single wire at distance R from it is:
$E(R)=\lambda/(2\pi \epsilon0*R)$
Finally, for two wires (one with positive charge and one with negative charge) having a distance $d=2a$ between them the total field is:
$E(R)=\lambda/(2\pi \epsilon0*R)+\lambda/(2\pi \epsilon0(d-R))=\lambda/(2\pi \epsilon0*R)+\lambda/(2\pi \epsilon0(2a-R))$
Potential difference
b)
To compute the potential difference we consider again the field of one wire
$V=\int_r0^{d=2a} \lambda/(2\pi \epsilon0*R) dR=[\lambda/(2\pi\epsilon0)]*ln(d/r0)=[\lambda/(2\pi\epsilon0)]*ln(2a/r0)$
Because there are two wires in the system (each having opposite charge) the actual potential difference is double this value. As a result:
$V=\lambda/(\pi\epsilon0)*ln(2a/r0)$
Capacitance
c)
The capacitance is by definition equal to total charge over the potential:
$C=Q/V$
Per unit length we have thus:
$C0=\lambda/V=(\pi\epsilon0)/ln(2a/r0)$
d)
If we are given the potential difference V we have:
$\lambda=(\pi\epsilon0*V)/ln(2a/r0)$
Back in the expression of the total field of one wire we obtain for the electric field a value of :
$Eb=\lambda/[2\pi\epsilon0*2a*ln(2a/r0)]$
Because there are two wires the field is actually double:
$Eb=V/(2a*ln(2a/r0))$
This is an equation that has to be solved for distance between wires $d=2a$.

